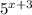Question

Simplifying/solving with common bases?

Answer 1

Given:

The given expression is
`5^(3 x) \cdot 5^(x+4) \cdot 5^(-3 x-1)`

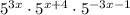
We need to simplify the given expression.

Simplification:

Let us use the exponent rule to simplify the given expression.

Let us apply the exponent rule,
`a^(b) \cdot a^(c)=a^(b+c)`

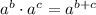
Thus, we have;

`5^(3 x) \cdot 5^(x+4) \cdot 5^(-3 x-1)=5^(3 x+x+4-3 x-1)`

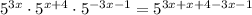
Now, we shall simplify the numerator.

The numerator of the expression can be simplified by adding the like terms.

Thus, we have;

`5^(3 x) \cdot 5^(x+4) \cdot 5^(-3 x-1)=5^(x+3)`

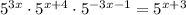
Thus, the simplified value of the given expression is
`5^(x+3)`