Question

Two wheel gears are connected by a chain. The larger gear has a radius of 5 centimeters and the

smaller gear has a radius of 3 centimeters. The smaller gear completes 36 revolutions in 20

seconds.

What is the linear velocity of each of the gears in centimeters per minute

Answer 1

The center velocity of both gears is same & equal to 2034.72
`(cm)/(min)`

Explanation:

Given data

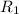`R_1` = 5 cm

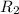`R_2` = 3 cm

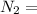`N_2 =` 36 rev in 20 sec

`\omega_2 = 2 \pi N_2`

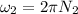
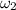`\omega_2` = 2 × 3.14 × 1.8

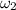`\omega_2` = 11.304
`(rad)/(s)`

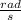
We know that for the set of gears

`R_1\omega_1 = R_2\omega_2`

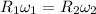
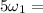`5 \omega_1 =` 3 × 11.304

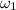`\omega_1` = 6.7824
`(rad)/(s)`

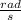
liner velocity of the first gear

`V_1 = R_1 \omega_1`

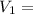`V_1 =` 5 × 6.7824

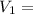`V_1 =` 33.912
`(cm)/(s)`

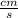
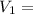`V_1 =` 20.34.72
`(cm)/(min)`

liner velocity of the second gear

`V_(2) = R_2 \omega_2`

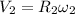
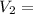`V_2 =` 3 × 11.304

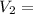`V_2 =` 2034.72
`(cm)/(min)`

Therefore the center velocity of both gears is same & equal to 2034.72
`(cm)/(min)`