Question

Pls help this is due today

Answer 1

The first step that we must take before attempting to solve the problem is to understand what the problem is asking us to do and what is given to us to help solve the problem. In this case, we are asked to solve for the area and we are provided with a figure that has 3 different corresponding lengths.

Moving onto the next step, we must determine what the area of the shape is. Looking at the shape, we can see as if there is a square and a triangle that have been glued together so we can find the area of each individual shape and add them up together to achieve the total area. Let us first determine the area of the square.

Area of the square

• 
  `A_(square) = side^2`

• 
  `A_(square) = (8\ yd)^2`

• 
  `A_(square) = (8)^2 * (yd)^2`

• 
  `A_(square) = 64\ yd^2`

Now that we have the area of the square finished, we can move onto the next part and determine the area of the triangle.

Area of the triangle

• 
  `A_(triangle) = (height\ *\ base)/(2)`

• 
  `A_(triangle) = (4\ yd\ *\ 8\ yd)/(2)`

• 
  `A_(triangle) = (32\ yd^2)/(2)`

• 
  `A_(triangle) = 16\ yd^2`

We have finally gotten the area for both the square and the triangle which means that we can add them together and determine the total area of the figure.

Total area of the figure

`A_(figure) = A_(square) + A_(triangle)`

`A_(figure) = 64\ yd^2 + 16\ yd^2`

`A_(figure) = 80\ yd^2`

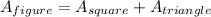
Therefore, after finding the area for both the triangle and square aspect of the figure we were able to combine them and get the total area of the figure which turned out to be 80 yards squared.