Answer:
The perimeter of Δ ABC is 20 + 2
 units ⇒ Last answer
units ⇒ Last answer
Explanation:
The perimeter of any triangle is the sum of the lengths of its three sides
The formula of distance between two points is
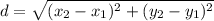
In Δ ABC
∵ A = (3 , 4) , B = (-5 , -2) , C = (5 , -2)
∵ AB = 10 units
∵ AC = 2

- To find its perimeter find the length of BC
∵
 = -5 and
= -5 and
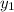 = -2
= -2
∵
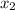 = 5 and
= 5 and
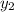 = -2
= -2
- By using the formula above
∴
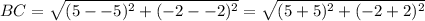
∴
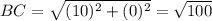
∴ BC = 10 units
To find the perimeter add the lengths of the three sides
∵ P = AB + BC + AC
∴ P = 10 + 10 + 2

- Add like terms
∴ P = 20 + 2

The perimeter of Δ ABC is 20 + 2
 units
units