Question

A survey of

400
400400 randomly selected homes from a large city with over
30
,
000
30,00030, comma, 000 homes showed that
16
1616 of the sampled homes didn't have a television.
Based on this sample, which of the following is a
95
%
95%95, percent confidence interval for the proportion of homes in this city that don't have a television?

Answer 1

The data is a random sample from the population of interest.

Individual observations can be considered independent.

Explanation:

Khan

Answer 2

0.0208<p<0.0592

Explanation:

-Given the sample size is 400 and the desired proportion is 16.

-The confidence interval can be determined as follows:

`CI=\hat p\pm z\sqrt{(\hat p(1-\hat p)/(n)}\\\\\hat p=x/n=16/400=0.04`

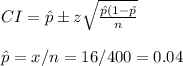
#We the use this proportion to find the CI at 95%:

`CI=0.04\pm 1.96* \sqrt{(0.04(1-0.04))/(400)}\\\\=0.04\pm 0.0192\\\\=[0.0208,0.0592]`

Hence, the 95% confidence interval is 0.0208<p<0.0592