The volume of composite figure is 385.17 cubic centimeters, if a half sphere and cone have a radius of 4 centimeters and height of 15 centimeters.
Explanation:
The given is,
Composite figure is combination of a half sphere and cone
Both have a radius of 4 centimeters
The cone has a height of 15 centimeters
Step:1
Volume of composite figure = Volume of half sphere + Volume of cone
Step:2
Formula for volume of semi sphere,

Where, r - Radius of Half sphere,
From given, r = 4 centimeters
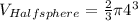
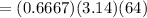 (∵
(∵
 = 3.14 )
= 3.14 )
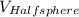 = 133.9733 cubic centimeters
= 133.9733 cubic centimeters
Formula for volume of cone,
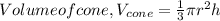
where, r - Radius of cone
h - Height of cone
From given,
r = 4 centimeters
h = 15 centimeters
Volume of cone,
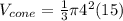
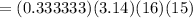 (∵
(∵
 = 3.14 )
= 3.14 )
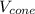
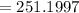 cubic centimeters
cubic centimeters
Step:3
Volume of composite figure = 133.9733 + 251.1997
Volume of composite figure = 385.17 cubic centimeters
Result:
The volume of composite figure is 385.17 cubic centimeters, if a half sphere and cone have a radius of 4 centimeters and height of 15 centimeters.