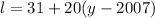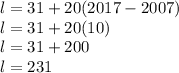Question

In 2007, there were 31 laptops

at Hawfields Middle. Starting

in 2008, the school purchased

20 more laptops at the end of

each year. Write the equation

to model this situation and find

the number of laptops at

Hawfields at the end of 2017.

Answer 1

`l=31+20(y-2007)`

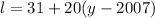
where
`l` is the number of laptops, and
`y` is the year.

in 2017:
`l=231`

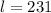
Explanation:

I will define the variable
`x` as the number of years that passed since 2007.

Since the school buys 20 lapts each year, after a number
`x` of years, the school will have

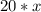`20*x` more laptops.

and thus, since the school starts with 31 laptops, the equation to model this situation is

`l=31+20*x`

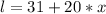
where
`l` is the number of laptops.

since x is the number of years that have passed since 2007, it can be represented like this:

`x=y-2007`

where
`y` can be any year, so the equation to model the situation using the year:

`l=31+20(y-2007)`

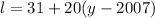
and this way we can find the number of laptos at the end of 2017:

`y=2017`

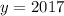
and

`l=31+20(y-2007)`

`l=31+20(2017-2007)\\l=31+20(10)\\l=31+200\\l=231`