Let the given complex number
z = x + ix =
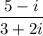
We have to find the standard form of complex number.
Solution:
∴ x + iy =
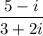
Rationalising numerator part of complex number, we get
x + iy =
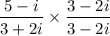
⇒ x + iy =

Using the algebraic identity:
(a + b)(a - b) =
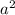 -
-

⇒ x + iy =
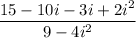
⇒ x + iy =
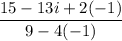 [ ∵
[ ∵
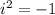 ]
]
⇒ x + iy =
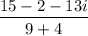
⇒ x + iy =
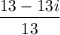
⇒ x + iy =
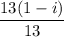
⇒ x + iy = 1 - i
Thus, the given complex number in standard form as "1 - i".