Question

A tennis ball is dropped from a height of 80 feet. After the first bounce, the ball reaches a height of 48 feet. After the second bounce, the ball reaches a height of 28.8 feet. After the third bounce, the ball reaches a height of 17.3 feet. Each time the ball hits the ground it only bounces up to 60% of the original height. By what factor is the ball's height changing after each bounce?

Answer 1

The factor is 0.60

Explanation:

we know that

The expression that model this situation is a exponential equation of the form

`h=a(b^x)`

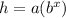
where

h is the height of the ball in feet

x is the number of bounces

a is the initial height

b is the factor

we have

`a=80\ ft\\b=60\%=60/100=0.60`

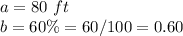
so

`h=80(0.60^x)`

Verify

First bounce

For x=1

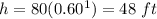`h=80(0.60^1)=48\ ft` ---> is ok

Second bounce

For x=2

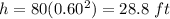`h=80(0.60^2)=28.8\ ft` ---> is ok

Third bounce

For x=3

`h=80(0.60^3)=17.28\ ft` ---> is ok

therefore

The factor is 0.60