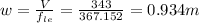Question

A high speed train is traveling at a speed of 44.7 m/s (100 mph) when the engineer sounds the 415 Hz warning horn. The speed of sound is 343 m/s. What are the frequency and wavelength of the sound, as perceived by a person standing at a crossing when the train is (a) approaching and (b) leaving the crossing

Answer 1

a) When the train is approaching the frequency is 477.19 Hz and the wavelenght is 0.719 m

b) When the train is leaving the crossing the frequency is 367.152 Hz and the wavelenght is 0.934 m

Step-by-step explanation:

Given data:

v = speed of the train = 44.7 m/s

V = speed of the sound = 343 m/s

f = frequency of sound = 415 Hz

a) If the train is approaching, the frequency is:

`f_(ap) =f((V)/(V-v) )=415((343)/(343-44.7) )=477.19Hz`

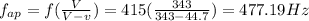
The wavelength is:

`w=(V)/(f_(ap) ) =(343)/(477.19) =0.719m`

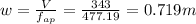
b) If the train leaves the crossing, the frequency is:

`f_(le) =f((V)/(V+v) )=415((343)/(343+44.7) )=367.152Hz`

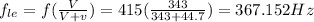
The wavelength is:

`w=(V)/(f_(le) ) =(343)/(367.152) =0.934m`