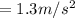Question

Europa, a satellite of Jupiter, is believed to have an ocean of liquid water (with the possibility of life) beneath its icy surface. Europa is 3130 km in diameter and has a mass of 4.78×10²² kg. In the future, we will surely want to send astronauts to investigate Europa.

In planning such a future mission, what is the fastest that such an astronaut could walk on the surface of Europa if her legs are 0.960 m long?

Answer 1

1.12 m/s

Step-by-step explanation:

We are given that

Diameter of Europa=d=3130 Km

Radius of Europa =
`r=(d)/(2)=\fra{3130}{2}=1565 Km=1565* 10^3 m`

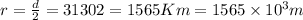
1 km=
`10^3 m`

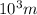
Mass of Europa,m=
`4.78* 10^(22) kg`

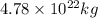
We have to find the velocity of astronaut on the surface of Europa if her legs are 0.960 m long.

d=0.960 m

Acceleration due to gravity on the surface of Europa

`g=(GM)/(r^2)`

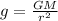
Where
`G=6.67* 10^(-11)Nm^2/kg^2`

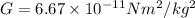
Substitute the values

`a=(6.67* 10^(-11)* 4.78* 10^(22))/((1565* 10^3)^2)`

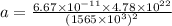
g
`=1.3 m/s^2`

`v=√(gd)=√(1.3* 0.960)=1.12 m/s`