Given question is incomplete. The complete question is as follows.
n-butane and isobutane are in equilibrium at 25 degrees centigrade.
n-butane(g)
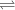 isobutane(g)
isobutane(g)
A one liter flask with the two species at equilibrium contains 0.170 mole isobutane and 0.0680 mole n-butane. Then the concentration of n-butane is increase by 0.200 moles. (0.200 mole n-butane is added.) What is the new equilibrium concentration for isobutane.
Step-by-step explanation:
As the given reaction is as follows.
n-butane(g)
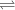 isobutane(g)
isobutane(g)
Initial: x 0
Equilbm: x - 0.17 0.17
It is given that equilibrium concentration of n-butane is 0.068 M.
So, x - 0.17 = 0.0680
x = 0.238
Therefore, the initial concentration is 0.238.
Here,
![K_(c) = ([isobutane])/([n-butane])](https://img.qammunity.org/2021/formulas/chemistry/college/fg3tn8h07hlwf1bmng9etwdbqyeq9ba1j0.png)
=
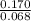
= 2.5
When 0.2 mol of n-butane is added. Hence, total moles will be as follows.
Total mole = (0.238 + 0.2)
= 0.438 mol
Hence, for 1 L volume the ICE table will be as follows.
n-butane(g)
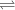 isobutane(g)
isobutane(g)
Initial: 0.438 0
Equilbm: 0.438 - x x
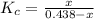
2.5 =
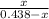
1.095 - 2.5x = x
x =
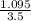
= 0.3128 M
Thus, we can conclude that the equilibrium concentration is 0.3128 M.