Question

Suppose that we want to estimate the mean cholesterol level in adults aged 55 or more. We choose a random sample of such levels. The random sample we choose has a mean of 197.5mg/dL and a standard deviation of 18.5mg/dL. For each of the following sampling scenarios, determine which test statistic is appropriate to use when making inference statements about the population mean.

Answer 1

If we analyze the size for the sample is < 30 and we don't know the population deviation so is better apply a t test to compare the actual mean to the reference value, and the statistic is given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

t-test: "Is used to compare group means. Is one of the most common tests and is used to determine if the mean is (higher, less or not equal) to an specified value".

b) t

Explanation:

We assume the following rest of the question

(Z refers to a variable having a standard normal distribution, and t refers to a variable having a t distribution.)

Sampling Scenario:

The sample has size 13, and it is from a normally distributed population with unknown standard deviation.

Determine which test statistic is appropriate to use:

a) Z

b) t

c) could use either Z or t

d) unclear

Solution to the problem

Data given and notation

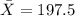`\bar X=197.5` represent the sample mean

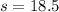`s=18.5` represent the sample standard deviation

`n=13` sample size

`\mu_o` represent the value that we want to test

`\alpha` represent the significance level for the hypothesis test.

t would represent the statistic (variable of interest)

`p_v` represent the p value for the test (variable of interest)

State the null and alternative hypotheses.

We need to conduct a hypothesis in order to check if the mean is (lower/higher or not equal) to an specified value, the system of hypothesis would be:

Null hypothesis:
`\mu = \mu_o`

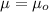
Alternative hypothesis:
`\mu \\eq \mu_o`

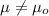
If we analyze the size for the sample is < 30 and we don't know the population deviation so is better apply a t test to compare the actual mean to the reference value, and the statistic is given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

t-test: "Is used to compare group means. Is one of the most common tests and is used to determine if the mean is (higher, less or not equal) to an specified value".

b) t