Answer:
The tensile stress on the wire is 550 MPa.
Step-by-step explanation:
Given;
Radius of copper wire, R = 3.5 mm
extension of the copper wire, e = 5.0×10⁻³ L
L is the original length of the copper wire,
Young's modulus for copper, Y = 11×10¹⁰Pa.
Young's modulus, Y is given as the ratio of tensile stress to tensile strain, measured in the same unit as Young's modulus.
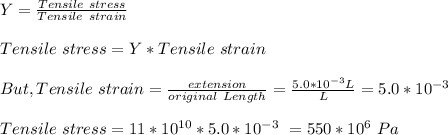
Therefore, the tensile stress on the wire is 550 MPa.