Answer:
The area of he field that is not reached by the sprinkler = 168.56

Explanation:
Side of the square field (a) = 28 m
Diameter of circular area (D) = 28 m
Radius of circular area (r)= 14 m
Area made by square field
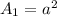
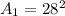 = 784
= 784

Area made by circular field
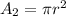
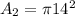
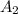 = 615.44
= 615.44

Field is not reached by the sprinkler A' =
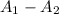
Put the values of
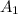 &
&
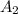
A' = 784 - 615.44
A' = 168.56

Therefore the area of he field that is not reached by the sprinkler = 168.56
