Answer:
a) mm
b) mm (of leaf width)/mm (of rain)
Explanation:
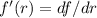
This represents the first derivative.
Phisically, represents the rate of variation of the leaf width respect to the rate of variation of the annual rainfall.
The independent variable is r, which units are mm of rain. The year is not needed as the variable is explicitly named "average annual rainfall".
The derivative has units of (mm of leaf)/(mm of rain), as it can be approximated as the division of this two differentials:
![[f'(r)]=[df/dr]=([\Delta w])/([\Delta r]) =(mm \,leaf)/(mm\,rain)](https://img.qammunity.org/2021/formulas/mathematics/college/a8hjo2wmpif7uilso51oviqwvl2ox5rptd.png)