Answer:
Therefore the population will be maximum when x=30 inches.
Explanation:
Given that, the mosquito population is a function of rainfall and can be approximated by the formula
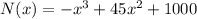
where x is the number of inches of rainfall.
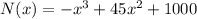
Differentiating with respect to x
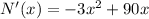
Again differentiating with respect to x

Now we set N'(x)=0
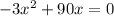
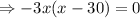
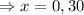
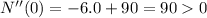
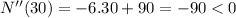
Since at x=30, N''(x)<0, So at x=30, N(x) has maximum value.
Therefore the population will be maximum when x=30 inches.