Answer:

d. -Sample selection was random
-Individual observations are independent of each other
np≥10
Explanation:
a. The point estimate of a sample proportion is obtained using the formula;

Hence, the point estimate of the proportion of the population is 0.2877
b. The desired margin of error is the calculated using the point estimate value as follows:
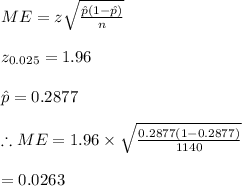
Hence, the desired margin of error for the sample proportion is 0.0263
c. Given a confidence level of 95%, the confidence interval can be calculated as:
![CI_(95\%)=\hat p\pm ME\\\\=0.2877\pm 0.0263\\\\=[0.2614,0.3140]](https://img.qammunity.org/2021/formulas/mathematics/college/8214qhdo7g7r3mz19n8rqkie1egv0xrr2x.png)
Hence, the confidence interval at 95% confidence level is 0.2614<p<0.3140
#We are 95% confident that the interval estimate contains the desired proportion.
d. The assumptions are:
-The sample size is is more than 10 or equal to 10:
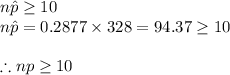
-The selection was from a randomized experiment.
-The individual observations were independent of each other.