Question

An agronomist wishes to estimate, to within one percentage point, the proportion of a new variety of seed that will germinate when planted, with 95% confidence. A typical germination rate is 97%. Estimate the minimum size sample required.

Answer 1

The minimum sample size must be 1118 to have margin of error within one percentage point.

Explanation:

We are given the following in the question:

Germination rate = 97% = 0.97

`\hat{p} = 0.97`

Margin of error = 1% = 0.01

We have to find the minimum sample size for a 95% confidence interval.

Formula for margin of error:

`z_(stat)* \sqrt{\frac{\hat{p}(1-\hat{p})}{n}}`

`z_(critical)\text{ at}~\alpha_(0.05) = 1.96`

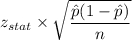
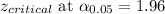
Putting values, we get,

`1.96* \sqrt{(0.97(1-0.97))/(n)}\leq 0.01\\\\√(n) \geq 1.96* (√(0.97(1-0.97)))/(0.01)\\\\√(n)\geq 33.4350\\\Rightarrow n \geq 1117.9056`

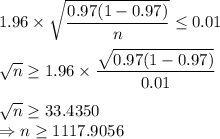
Rounding off to integer,

`n = 1118`

Thus, the minimum sample size must be 1118 to have margin of error within one percentage point.