Answer:
Part A) The average rate of change of section A is 6 and the average rate of change of section B is 24
Part B) The average rate of change of Section B is 4 times greater than the average rate of change of Section A. see the explanation
Explanation:
Part A) Find the average rate of change of each section
we know that
To find the average rate of change, we divide the change in the output value by the change in the input value
the average rate of change is equal to

In this problem we have
Section A
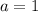
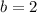
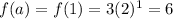

Substitute

Section B
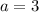
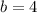
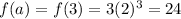
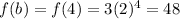
Substitute

Part B) How many times greater is the average rate of change of Section B than Section A? Explain why one rate of change is greater than the other
Divide the average rate of change section B by the average rate of change of Section B
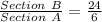
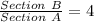
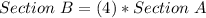
so
The average rate of change of Section B is 4 times greater than the average rate of change of Section A.
One rate of change is greater than the other, because it is an exponential function and the slope grows bigger.