Answer:
a. OT
b. 9.9*10^{-7}T
c. 4.6*10^{-7}T
Step-by-step explanation:
The strength of a magnetic field generated by a current in a wire is
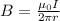
where mu0 is the magnetic permeability, I is the current and r is the distance in which we want to know the magnitude of B.
a. midway between the wires
r1=0.25m
r2=0.25cm
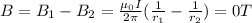
b. at a point in the plane of the wires but 3.00 m from the nearer of the two
r1=3.5m
r2=3m
in this case we have
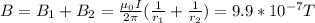
c. at a point between and above the two wires, 1.01 m from each one
- between the wires, as in the point a B=0T
- above each wire:
r1=1.01m
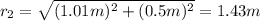
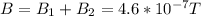
HOPE THIS HELPS!!