Answer:
The rate at which water is being pumped into the tank is 13,913.27 cubic centimetre per minute
Explanation:
Given that, the height of the conical tank is 13 meters and diameter is 3 meters.
Radius =
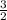 meters
meters
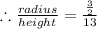

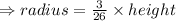 ......(1)
......(1)
The volume of the cone is
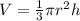
Now putting
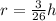
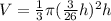

Differentiating with respect to t
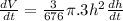
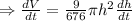
Given that, the water level is rising at a rate of 17 cm per minute when the height 1 meter= 100 cm .i.e
 cm/min
cm/min
Putting


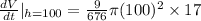
≈7,113 cubic centimetre per minute
The volume of water increases 7,113 cubic centimetre per minute while 6,800 cubic centimetre per minute is leaking out.
It means the required rate at which water is being pumped into the tank is
=(7,113+6,800)cubic centimetre per minute
=13,913 cubic centimetre per minute