Question

he exponential model Upper A equals 347.5 e Superscript 0.024 t describes the​ population, A, of a country in​ millions, t years after 2003. Use the model to determine when the population of the country will be 510 million.

Answer 1

The population of the country will be 510 million during the year of 2018.

Explanation:

The exponential model for the population, in millions, t years after 2003 is.

`P(t) = 347.5e^(0.024t)`

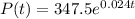
Use the model to determine when the population of the country will be 510 million.

t years after 2003, in which t is found when P(t) = 510. So

`P(t) = 347.5e^(0.024t)`

`510 = 347.5e^(0.024t)`

`e^(0.025t) = (510)/(347.5)`

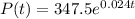
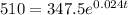
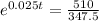
Applying ln to both sides, so we can find t

`\ln{e^(0.025t)} = \ln{(510)/(347.5)}`

`t = \frac{\ln{(510)/(347.5)}}{0.025}`

`t = 15.35`

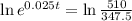
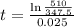
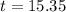
15.35 years after 2003, so during the year of 2018.

The population of the country will be 510 million during the year of 2018.