Question

The probability that person A will pass Finite Mathematics is 7/8 and the probability that person B will pass is 4/7 . Assume the events are independent. Find the probability that person A will fail given that person B will pass.

Answer 1

0.1429 is the required probability.

Explanation:

We are given the following in the question:

The probability that person A will pass Finite Mathematics =

`P(A)=(7)/(8)`

`P(A') = (1)/(8)`

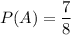
The probability that person A will pass Finite Mathematics =

`P(B)=(4)/(7)`

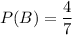
The two events are independent.

We have to find the conditional probability that person A will fail given that person B will pass.

`P(A'|B)=(P(A'\cap B))/(P(B))\\\\\text{Since A and B are independent events,}\\\\ = (P(A')* P(B))/(P(B))=(1)/(7) = 0.1429`

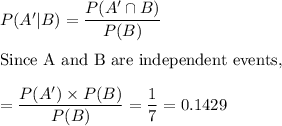
Thus, 0.1429 is the probability that person A will fail given that person B will pass.