Question

Imagine that researchers appropriately conduct a paired-samples t test with 25 pairs of related scores. The mean difference between those 25 pairs of scores was 5.00. The standard deviation for the 25 difference scores was 7.50. What is the corresponding value for Cohen's d (rounded to three decimal places)? When computing your answer, please assume that the researchers are using a standard null hypothesis that predicts no differences.

Answer 1

Cohen's d = 0.667

Explanation:

We are given the following in the question:

A paired sample t-test is conducted.

Sample size, n = 25

The mean difference between 25 pairs = 5.00

`M_1-M_2 = 5.00`

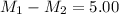
where
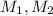`M_1,M_2` are the means for paired t-test.

The standard deviation for the 25 difference scores = 7.50

`S.D_(Pooled) = 7.50`

Formula for Cohen's d:

`=(M_1-M_2)/(S.D_(Pooled))`

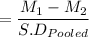
Putting values, we get,

Cohen's d =

`=(5.00)/(7.50)\\\\=0.667`

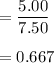
The value of Cohen's d coefficient is 0.667