Question

According to a candy​ company, packages of a certain candy contain 1616​% orange candies. Suppose we examine 100100 random candies. a. What value should we expect for our sample percentage of orange​ candies? b. What is the standard​ error? c. Use your answers to fill in the blanks below. We expect​ ____% orange​ candies, give or take​ _____%.

Answer 1

a) By the Central Limit Theorem, 16%.

b) 0.0367 = 3.67%

c) We expect 16% orange​ candies, give or take 3.67%.

Explanation:

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

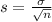For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

For samples of size n of a proportion p, the expected sample percentage is p and the standard error is
`s = \sqrt{(p(1-p))/(n)}`

In this problem, we have that:

`p = 0.16, n = 100`

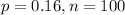
a. What value should we expect for our sample percentage of orange​ candies?

By the Central Limit Theorem, 16%.

b. What is the standard​ error?

`s = \sqrt{(0.16*0.84)/(100)} = 0.0367`

0.0367 = 3.67%

c. Use your answers to fill in the blanks below. We expect​ ____% orange​ candies, give or take​ _____%.

We expect 16% orange​ candies, give or take 3.67%.