Question

What connection(s) can you make between the Quotient Rule and Zero as an Exponent Rule?

What connection(s) can you make between the Quotient Rule and the Negative Exponent Rule?

Answer 1

Explanation:

For any nonzero real number a , the zero exponent rule of exponents states that
`a^(0) = 1`

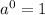
In this case, we would use the zero exponent rule of exponents to simplify the expression to 1 . To see how this is done, let us begin with an example.

`(t^(8) )/(t^(8) ) = 1`

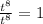
If we were to simplify the original expression using the quotient rule, we would have

`(t^(8) )/(t^(8) ) = t^(8-8) = t^(0)`

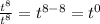
If we equate the two answers, the result is
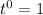`t^(0) =1` . This is true for any nonzero real number, or any variable representing a real number.

`a^(0)=1`

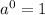
The sole exception is the expression
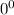`0^(0)` . This appears later in more advanced courses, but for now, we will consider the value to be undefined.