Answer:
Therefore the towns are apart 225 miles.
Explanation:
Given that, Donna travels from one town to another town at a speed of 45 mph.
Assume that, x be the distance that was traveled by Donna .
We know that,
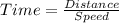
So, Donna takes
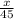 hours for the tripe.
hours for the tripe.
She could've made the trip in a half an hour less time,if she traveled 5 mph faster,
Now she could made the trip in
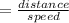
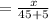
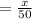 hours.
hours.
According to the problem,
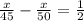
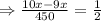
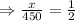
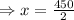
 miles
miles
Therefore the towns are apart 225 miles.