Answer: (82.66, 87.34).
Explanation:
When population standard deviation is unknown and sample size is small , then the formula is used to find the confidence interval for
 is given by :-
is given by :-
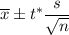
, where n = sample size ,
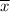 = sample mean , t*= two tailed critical value s= sample population standard deviation, .
= sample mean , t*= two tailed critical value s= sample population standard deviation, .
Given,
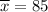 , s=5, n=20 , degree of freedom = 19 [∵df=n-1]
, s=5, n=20 , degree of freedom = 19 [∵df=n-1]
For 95% confidence level ,

By t-distribution table ,
t-value for
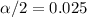 (two tailed) and df =19 is t*=2.0930
(two tailed) and df =19 is t*=2.0930
Now , the 95% confidence interval for the mean heart rate of adults in the population will be :
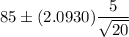
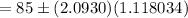
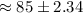
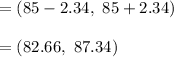
Hence, the required interval is (82.66, 87.34).
Interpretation : A person can be 95% confident that the mean heart rate of adults in the population lies between (82.66, 87.34).