Answer:
a) n= 1045 computers
b) n= 442 computers
c) A. Yes, using the additional survey information from part (b) dramatically reduces the sample size.
Explanation:
Hello!
The variable of interest is
X: Number of computers that use the new operating system.
You need to find the best sample size to take so that the proportion of computers that use the new operating system can be estimated with a 99% CI and a margin of error no greater than 4%.
The confidence interval for the population proportion is:
p' ±
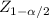 *
*
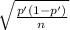
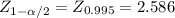
a) In this item there is no known value for the sample proportion (p') when something like this happens, you have to assume the "worst-case scenario" that is, that the proportion of success and failure of the trial are the same, i.e. p'=q'=0.5
The margin of error of the interval is:
d=
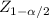 *
*
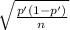

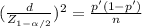
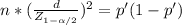
![n= [p'(1-p')]*((Z_(1-\alpha /2))/(d) )^2](https://img.qammunity.org/2021/formulas/mathematics/college/sjdmlb8lldqy4flonrtu332d5actyx0izz.png)
![n=[0.5(1-0.5)]*((2.586)/(0.04) )^2= 1044.9056](https://img.qammunity.org/2021/formulas/mathematics/college/jywtp5cfq1333so37ace5n65qeu3kb4cws.png)
n= 1045 computers
b) This time there is a known value for the sample proportion: p'= 0.88, using the same confidence level and required margin of error:
![n= [p'(1-p')]*((Z_(1-\alpha /2))/(d) )^2](https://img.qammunity.org/2021/formulas/mathematics/college/sjdmlb8lldqy4flonrtu332d5actyx0izz.png)
![n= [0.88*0.12]*(\frac{{2.586}}{0.04})^2= 441.3681](https://img.qammunity.org/2021/formulas/mathematics/college/p4zbavzao1mu071orkcgqw5x4ahktxfg4l.png)
n= 442 computers
c) The additional information in part b affected the required sample size, it was drastically decreased in comparison with the sample size calculated in a).
I hope it helps!