Answer:
The separation between third order and first order is 0.0304 m
Step-by-step explanation:
Given:
Separation between two slit
 m
m
Distance between slit and screen
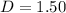 m
m
Wavelength of light
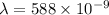 m
m
From the formula of interference of light,
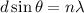
Here
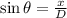
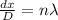
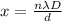
Where
 separation between fringes
separation between fringes
Here we have to find between third order and first order,
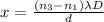
Where
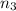 = 3
= 3
 = 1
= 1
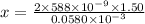
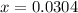 m
m
Therefore, the separation between third order and first order is 0.0304 m