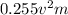Question

A car is moving with a constant speed v around a level curve. The coefficient of friction between the tires and the road is 0.40. What is the minimum radius of curve if the car is to stay on the road?

Answer 1

Minimum radius of the curve to stay on the road will be equal to
`0.255v^2m`

Step-by-step explanation:

We have given coefficient of kinetic friction
`\mu =0.40`

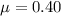
Acceleration due to gravity
`g=9.8m/sec^2`

We have to find minimum radius of the curve so that car will stay on the road

For the car to stay on the road centripetal force must be equal to frictional force

So
`(mv^2)/(r)=\mu mg`

So minimum radius of the

`r=(v^2)/(\mu g)=(v^2)/(0.4* 9.8)=0.255v^2m`

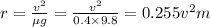
So minimum radius of the curve to stay on the road will be equal to
`0.255v^2m`