Question

After arriving at the university student medical clinic, the waiting times to receive service after checking-in follow an exponential distribution with a mean of 10 minutes.

How many students are served per hour?



Calculate the probability a student waits less than 6 minutes.

Answer 1

6 students are served per hour.

45.12% probability a student waits less than 6 minutes.

Explanation:

Exponential distribution:

The exponential probability distribution, with mean m, is described by the following equation:

`f(x) = \mu e^(-\mu x)`

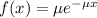
In which
`\mu = (1)/(m)` is the decay parameter.

The probability that x is lower or equal to a is given by:

`P(X \leq x) = \int\limits^a_0 {f(x)} \, dx`

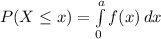
Which has the following solution:

`P(X \leq x) = 1 - e^(-\mu x)`

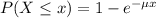
mean of 10 minutes.

This means that
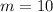`m = 10`, so
`\mu = (1)/(10) = 0.1`

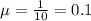
How many students are served per hour?

One student is served each 10 minutes, on average

An hour has 60 minutes

60/10 = 6

6 students are served per hour.

Calculate the probability a student waits less than 6 minutes.

`P(X \leq x) = 1 - e^(-0.1*6) = 0.4512`

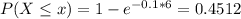
45.12% probability a student waits less than 6 minutes.