Answer:
Probability (at least 1 burgundy bead) =

Explanation:
Briefcase contains: 11 black beads; 9 burgundy beads
According to the question,
Ananya picked 2 beads without replacement;
Probability (at least 1 burgundy beads) = probability (1 burgundy beads) + probability (2 burgundy beads)
Now, we know that for any event,
probability (occurrence of event) + probability (non occurrence of event) = 1
so, the above case can also be rewritten as
Probability (at least 1 burgundy beads)= 1 - probability (no burgundy beads)
Probability (at least 1 burgundy beads)= 1 - probability (both black beads)
Probability (at least 1 burgundy beads) = 1 - probability (black, black)
Probability (at least 1 burgundy beads) = 1 - (
 )
)
Probability (at least 1 burgundy beads) = 1 -
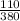
Probability (at least 1 burgundy beads) =
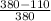
Probability (at least 1 burgundy beads) =
