Question

The overhead reach distances of adult females are normally distributed with a mean of 200 cm and a standard deviation of 8.9 cm. a. Find the probability that an individual distance is greater than 209.30 cm.

Answer 1

0.148 or 14.80%

Explanation:

Mean overhead reach (μ) = 200 cm

Standard deviation (σ) = 8.9 cm

The z-score for any reach, X, is given by:

`z=(X-\mu)/(\sigma)`

For a X = 209.30 cm:

`z=(209.3-200)/(8.9)\\ z=1.045`

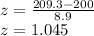
A z-score of 1.045 corresponds to the 85.20th percentile.

Therefore, the probability that an individual distance is greater than 209.30 cm is:

`P = 1-0.8520\\P=0.148=14.80\%`

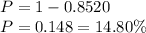
The probability is 0.148 or 14.80%