Parabola with focus (0,2) and directrix y=-1 is y=-x²+1.
To find an equation of the parabola whose graph is shown, we can use the following steps:
1. Identify the focus and directrix. The focus of a parabola is a point on the axis of symmetry that is equidistant from all points on the parabola. The directrix of a parabola is a line that is parallel to the axis of symmetry and is equidistant from all points on the parabola.
From the image, we can see that the parabola is symmetric about the y-axis, so the axis of symmetry is the y-axis. The focus is labeled as (0, 2) and the directrix is labeled as y = -1.
2. Determine the type of parabola. There are three types of parabolas: parabolas that open upward, parabolas that open downward, and parabolas that open to the side.
From the image, we can see that the parabola opens downward.
3. Write the equation of the parabola in vertex form. The vertex form of a parabola is given by the following equation:
y = A(x - h)² + k
where (h, k) is the vertex of the parabola and A is a constant that determines the shape of the parabola.
To find the vertex of the parabola, we can use the following steps:
1. Find the midpoint of the focus and the directrix. This will be the vertex of the parabola.
2. Substitute the coordinates of the vertex into the equation of the parabola to solve for A.
The midpoint of the focus and the directrix is (0, 1). Substituting this into the equation of the parabola, we get:
1 = A(0 - h)² + k
Since the parabola opens downward, A must be negative. Substituting A = -1, we get:
1 = -(h)² + k
Solving for k, we get:
k = 1 + (h)²
Therefore, the equation of the parabola in vertex form is:
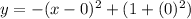
Simplifying the equation, we get:
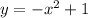
Therefore, the equation of the parabola whose graph is shown is
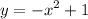 .
.