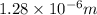Question

Line spectra from all regions of the electromagnetic spectrum, including the Paschen series of infrared lines for hydrogen, are used by astronomers to identify elements present in the atmospheres of stars. Calculate the wavelength of the photon emitted when the hydrogen atom undergoes a transition from n = 5 to n = 3. (R = 1.096776 × 107 m–1)

Answer 1

Answer : The wavelength of the photon emitted is,
`1.28* 10^(-6)m`

Explanation :

Using Rydberg's Equation:

`(1)/(\lambda)=R_H\left((1)/(n_i^2)-(1)/(n_f^2) \right )`

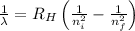
Where,

`\lambda` = Wavelength of radiation

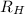`R_H` = Rydberg's Constant = 1.096776 × 10⁷ m⁻¹

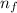`n_f` = Higher energy level = 5

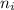`n_i`= Lower energy level = 3

Now put all the given values, in above equation, we get:

`(1)/(\lambda)=(1.096776* 10^7)\left((1)/(3^2)-(1)/(5^2) \right )`

`\lambda=1.28* 10^(-6)m`

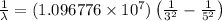
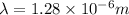
Therefore, the wavelength of the photon emitted is,
`1.28* 10^(-6)m`