Option 2.
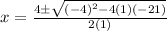 shows the correct way to use the quadratic formula to solve the given equation.
shows the correct way to use the quadratic formula to solve the given equation.
Explanation:
Step 1:
For an equation of the form
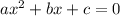 the solution is
the solution is
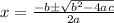 .
.
Here a is the coefficient of
 , b is the coefficient of x and c is the constant term.
, b is the coefficient of x and c is the constant term.
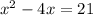 can also be written as
can also be written as

Comparing
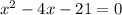 with
with
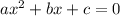 , we get that a is 1, b is -4 and c is -21.
, we get that a is 1, b is -4 and c is -21.
To get the solution, we substitute the values of a, b, and c in
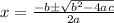 .
.
Step 2:
Substituting the values, we get
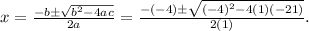
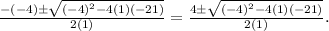
This is option 2.