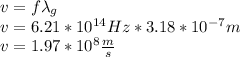Question

(a) What is the frequency of light with a 483 nm wavelength in air?

(b) What is its wavelength in glass with an index of refraction of 1.52?
(c) From the results of (a) and (b) find its speed in this glass.

Answer 1

(a) The light is a electromagnetic wave. The frequency of a wave is given by:

`f=(v)/(\lambda)`

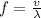
Where v is the speed of the wave and
`\lambda` its wavalength. In this case, v is the speed of light c.

`f=(c)/(\lambda)\\f=(3*10^8(m)/(s))/(489*10^(-9)m)\\f=6.21*10^(14)Hz`

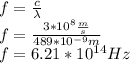
(b) The wavelength of light in glass is defined as:

`\lambda_g=(\lambda_a)/(n)\\\lambda_g=((483*10^(-9)m))/(1.52)\\\lambda_g=3.18*10^(-7)m`

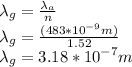
(c) According to the first equation, the speed of the light in this glass is:

`v=f\lambda_g\\v=6.21*10^(14)Hz*3.18*10^(-7)m\\v=1.97*10^(8)(m)/(s)`