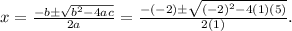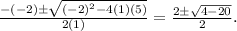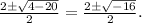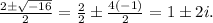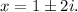Question

Use the quadratic formula to solve the equation x^2-2x+5=0

Answer 1

For the given equation, the solutions are
`x = 1 \pm 2i.`

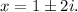
Explanation:

Step 1:

For an equation of the form
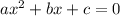`ax^(2) +bx+c=0` the solution is
`x=\frac{-b \pm \sqrt{b^(2)-4 a c}}{2 a}`.

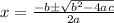Here a is the coefficient of
`x^(2)`, b is the coefficient of x and c is the constant term.

Comparing
`x^(2) -2x+5=0` with
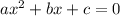`ax^(2) +bx+c=0`, we get that a is 1, b is -2 and c is 5.

To get the solution, we substitute the values of a, b, and c in
`x=\frac{-b \pm \sqrt{b^(2)-4 a c}}{2 a}`.

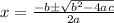Step 2:

Substituting the values, we get

`x=\frac{-b \pm \sqrt{b^(2)-4 a c}}{2 a}=\frac{-(-2) \pm \sqrt{(-2)^(2)-4(1)(5)}}{2(1)}.`

`\frac{-(-2) \pm \sqrt{(-2)^(2)-4(1)(5)}}{2(1)} = (2 \pm √(4-20))/(2).`

`(2 \pm √(4-20))/(2) = (2 \pm √(-16))/(2).`

`(2 \pm √(-16))/(2) = (2)/(2) \pm (4(-1))/(2) = 1 \pm 2i.`

`x = 1 \pm 2i.`