Question

HURRY 70 POINTS!! solve the system using substitution. show all work. {4x + 5y = 7 {y = 3x - 10

Answer 1

(3, -1)

Explanation:

Given

• 4x + 5y = 7
• y = 3x - 10

Subbing the value of y

• 4x + 5(3x - 10) = 7
• 4x + 15x - 50 = 7
• 19x = 57
• x = 3
• y = 3(3) - 10
• y = 9 - 10
• y = -1
• Solution is (3, -1)

Answer 2

• x = 3
• y = -1

Solution: (3, -1)

Explanation:

Given equations:

• 
  `4x + 5y = 7`
• 
  `y = 3x - 10`

Step-1: Reorganize the equations with "y" on the L.H.S

`\rightarrow \left \{ {{4x + 5y = 7} \atop {y = 3x - 10}} \right.`

`\rightarrow \left \{ {{5y = 7 - 4x} \atop {y = 3x - 10}} \right.`

`\rightarrow \left \{ {{5y = 7 - 4x} \atop y =-10 + 3x}} \right.`

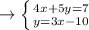
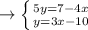
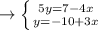
Step-2: Make the x-variables the same on both equations

`\rightarrow \left \{ {{3(5y = 7 - 4x)} \atop 4(y =-10 + 3x)}} \right.`

`\rightarrow \left \{ {{15y = 21 - 12x} \atop 4y =-40 + 12x}} \right.`

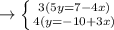
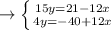
Step-3: Add the equations

`\rightarrow (15y + 4y) = (21 - 40) + (-12x + 12x)`

`\rightarrow 19y = -19`

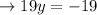
Step-4: Divide both sides by 19

`\rightarrow (19y)/(19) = (-19)/(19)`

`\rightarrow y = -1`

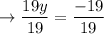
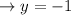
Step-5: Substitute the value of y into any equation

`\rightarrow y = 3x - 10`

`\rightarrow -1 = 3x - 10`

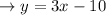
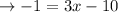
Step-6: Simplify the equation

`\rightarrow -1 + 10 = 3x`

`\rightarrow9= 3x`

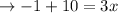
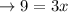
Step-7: Divide 3 both sides

`\rightarrow (9)/(3) = (3x)/(3)`

`\rightarrow x = 3`

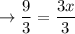
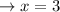
(Optional) Step-8: Convert the solution(s) into (x, y) form

`\rightarrow (x, y) \rightarrow (x = 3; y=-1) \rightarrow (3,-1)`

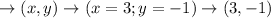
Thus, the value of x and y is 3 and -1. The solution is (3, -1).