Question

A multimeter in an RL circuit records an rms current of 0.600 A and a 50.0-Hz rms generator voltage of 110 V. A wattmeter shows that the average power delivered to the resistor is 14.0 W.

(a) Determine the impedance in the circuit.
(b) Determine the resistance, R.
(c) Determine the inductance, L.

Answer 1

(a) The impedance in the circuit is
`Z=183.33\Omega`.

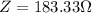(b)The resistance is
`R=38.89\Omega`.

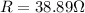(c) The inuctance is 0.57 H.

Step-by-step explanation:

(a)

The expression for the impedance is as follows:

`Z=(V_rms)/(I_rms)`

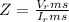
Here,
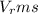`V_rms` is the rms voltage and
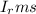`I_rms` is the rms current.

Put
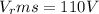`V_rms=110 V` and
`I_rms=0.600 A`.

`Z=(110)/(0.600)`

`Z=183.33\Omega`

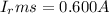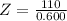
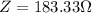
Therefore, the impedance in the circuit is
`Z=183.33\Omega`.

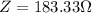(b)

The expression for the average power is as follows;

`P_(a)=I_(rms)^(2)R`

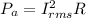
Here,
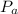`P_(a)` is the average power and R is the resistance.

Calculate the resistance by rearranging the above expression.

`R=(P_(a))/(I_(rms)^(2))`

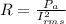
Put
`P_(a)=14W` and

`R=\frac{14}{{0.600}^(2)}`

`R=38.89\Omega`

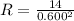
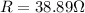
Therefore, the resistance is
`R=38.89\Omega`.

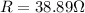(c)

The expression for the impedance is as follows;

`Z^(2)=R^(2)+X_(L)^(2)`

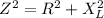
Here,
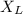`X_(L)` is the inductive reactance.

Put
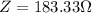`Z=183.33\Omega` and
`R=38.89\Omega`.

`(183.33)^(2)=(38.89)^(2)+X_(L)^(2)`

`X_(L)=179.16\Omega`

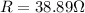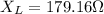
The expression for the inductive reactance in terms of frequency is as follows;

`X_(L)=2\pi fL`

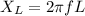
Here, L is the inductance.

Calculate the inductance by rearranging the above expression.

`L=(X_(L))/(2\pi f)`

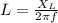
Put
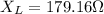`X_(L)=179.16\Omega` and f=50Hz.

`L=(179.16)/(2\pi (50))`

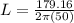
L=0.57 H

Therefore, the inuctance is 0.57 H.