Question

An individual who has automobile insurance from a certain company is randomly selected. Let Y be the number of mov- ing violations for which the individual was cited during the last 3 years. The pmf of Y is:

y 0 1 2 3
P(y) 0.50 0.25 0.20 0.05

a. Compute E(Y).
b. Suppose an individual with Y violations incurs a surcharge of $110Y2. Calculate the expected amount of the surcharge.

Answer 1

a) E(y) = 0.8

b) The average subcharge is $165

Explanation:

We are given the following distribution in the question:

y: 0 1 2 3

P(y): 0.50 0.25 0.20 0.05

a) E(y)

`E(y) = \displaystyle\sum y_iP(y_i)\\\\E(y) = 0(0.50)+1(0.25)+2(0.20)+3(0.05)\\E(y) = 0.8`

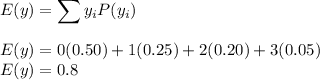
b) Expected value of subcharge

Subcharge =

`\$110y^2`

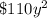
Expected value of subcharge =

`=110E(y^2)\\\\=110\displaystyle\sum y_i^2P(y_i)\\\\=110(0^2(0.50)+1^2(0.25)+2^2(0.20)+3^2(0.05))\\=110(1.5)=165`

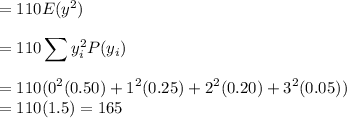
Thus, the average subcharge is $165