Answer:
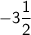
Solution:
- First, we need to flop the second fraction over.
- Then, we need to multiply the first fraction times the second fraction.
- In order to multiply fractions, we need to multiply the numerator of the first fraction times the numerator of the second fraction:
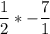

- Now, let's convert the improper fraction into a mixed number.
- First, how many times does 2 go into 7? (evenly)
- The answer is 3.
- Now, multiply 3 times 2. We get 6.
- Subtract 7-6. We get 1.
- The denominator stays the same.
- Therefore, the answer is
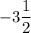
Hope it helps.
Do comment if you have any query.