Answer:
The option C) 15 s is correct
Therefore the maximum height it reaches in 15 seconds.
Explanation:
Given equation is

Given that a projectile is thrown upward so that its distance above the ground after t seconds is

To find how many seconds does it reach its maximum height:
"The standard form of a parabola's equation is expressed as :
 .
.
If
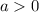 , then the parabola opens upwards;
, then the parabola opens upwards;
if
 the parabola opens downwards."
the parabola opens downwards."
The maximum height is the vertex of the parabola
 which is
which is
 .
.
Comparing the given equation with the standard form of parabola we get
the values of a=-13 , b=390 and c=0
The maximum height in
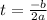 .
.
Substitute the values we get
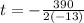
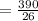
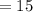
∴ t=15 s
∴ The option C) 15 s is correct.
∴ the maximum height is the vertex of the parabola, at t reaches in 15 seconds.