Question

10 points

4. Assume that segments that appear to be tangent are tangent. Enter
the number only for your answer.
If PQ = 4x + 2, QR = 7x – 19, and QU = 34, find ST.
Your answer

Answer 1

Given:

The lengths PQ = 4x + 2, QR = 7x – 19, and QU = 34

We need to determine the value of ST.

Value of x:

By two tangent theorem, we have;

PQ = QR

Substituting the values, we get;

`4x+2=7x-19`

`-3x+2=-19`

`-3x=-21`

`x=7`

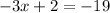
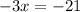
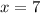
Thus, the value of x is 7.

Length of PQ and QR:

Substituting x = 7 in the expressions of PQ and QR, we get the lengths.

Length of PQ =
`4(7)+2=28+2=30`

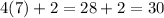
Length of QR =
`7(7)-19=49-19=30`

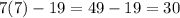
Radius of the circle:

The radius of the circle PU can be determined using the Pythagorean theorem.

Thus, we have;

`QU^2=PQ^2+PU^2`

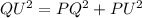
Substituting the values, we get;

`34^2=30^2+PU^2`

`1156=900+PU^2`

`256=PU^2`

`16=PU`

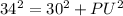
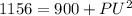
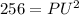
Thus, the radius of the circle is 16.

Length of ST:

The length of ST is
`ST=SU+UT`

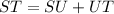
Substituting SU = UT = 16 because the radius of the circle is 16.

Thus, we have;

`ST=16+16`

`ST=32`

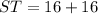
Thus, the length of ST is 32.