Question

Before beginning a long trip on a hot day, a driver inflates anautomobile tire to a gauge pressure of 2.70 atm at 300 K. At the end of the trip the gaugepressure has increased to 3.20 atm.

(a) Assuming that the volume has remainedconstant, what is the temperature of the air inside the tire?
1 K
(b) What percentage of the original mass of air in the tire shouldbe released so the pressure returns to the original value? Assumethe temperature remains at the value found in (a), and the volumeof the tire remains constant as air is released.

Answer 1

The value is the temperature of the air inside the tire
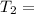`T_(2) =` 340.54 K

% of the original mass of air in the tire should be released 99.706 %

Step-by-step explanation:

Initial gauge pressure = 2.7 atm

Absolute pressure at inlet
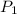`P_(1)` = 2.7 + 1 = 3.7 atm

Absolute pressure at outlet
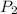`P_(2)` = 3.2 + 1 = 4.2 atm

Temperature at inlet
`T_(1)` = 300 K

(a) Volume of the system is constant so pressure is directly proportional to the temperature.

`(T_(2) )/(T_(1) ) = (P_(2) )/(P_(1) )`

`(T_(2) )/(300) = (4.2)/(3.7)`

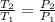
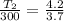
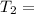`T_(2) =` 340.54 K

This is the value is the temperature of the air inside the tire

(b). Since volume of the tyre is constant & pressure reaches the original value.

From ideal gas equation P V = m R T

Since P , V & R is constant. So

m T = constant

`m_(1) T_(1) = m_(2) T_(2)`

`(m_(2) )/(m_(1) ) = (T_(1) )/(T_(2) )`

`(m_(2) )/(m_(1) ) = (300)/(354.54)`

`(m_(2) )/(m_(1) ) =0.00294`

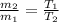
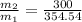
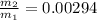
value of the original mass of air in the tire should be released is
`(m_(2) - m_(1))/(m_(1))`

`(0.00294-1)/(1)`

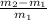
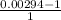
⇒ -0.99706

% of the original mass of air in the tire should be released 99.706 %.