Given:
The given equation is
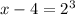
Solving the equation
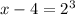 , we get;
, we get;
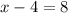
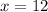
We need to determine the logarithmic equation that is equivalent to the given equation.
Option A:
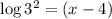
Simplifying, we get;
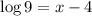

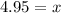
Since, the values of x are not equivalent, the equation
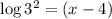 is not equivalent to
is not equivalent to
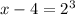
Option A is not the correct answer.
Option B:
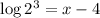
Simplifying, we get;
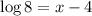

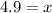
Since, the values of x are not equivalent, the equation
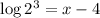 is not equivalent to
is not equivalent to
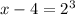
Option B is not the correct answer.
Option C:

Simplifying, we get;
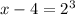
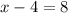
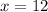
Since, the values of x are equivalent, the equation
 is equivalent to
is equivalent to
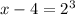
Hence, Option C is the correct answer.
Option D:

Simplifying, we get;


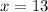
Since, the values of x are not equivalent, the equation
 is not equivalent to
is not equivalent to
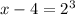
Hence, Option D is not the correct answer.