Question

On square PQRS below, if Q is located at

(7,0) and R is located at (5, -8), what is
the length of SR ?

Answer 1

2√17

Explanation:

Answer 2

the length of SR is
`2√(17)` .

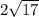Explanation:

Here we have , On square PQRS below, if Q is located at (7,0) and R is located at (5,-8) . We need to find Length of side SR . Let's find out:

We know that , In a square there are 4 sides , and length of every side is same . So side length of SR = side length of QR . Now , Let's find side length of QR .

We know that distance between two points
`Q(x_1,y_1),R(x_2,y_2)` given by :

⇒
`QR = √((x_2-x_1)^2+(y_2-y_1)^2)`

Two points given are
`Q(7,0), R(5,-8)` :

⇒
`QR = √(5-7)^2+(-8-0)^2)`

⇒
`QR = √((2)^2+(-8)^2)`

⇒
`QR = √(4+64)`

⇒
`QR = √(68)`

⇒
`QR = 2√(17)`

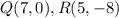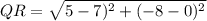
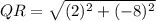
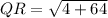
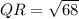
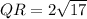
But QR=SR , Therefore , the length of SR is
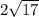`2√(17)` .