Question

A waiter believes the distribution of his tips has a model that is slightly skewed to the right​, with a mean of ​$10.50 and a standard deviation of ​$5.20. He usually waits on about 50 parties over a weekend of work. ​a) Estimate the probability that he will earn at least ​$600. ​b) How much does he earn on the best 1​% of such​ weekends?

Answer 1

(a) The probability that he will earn at least ​$600 is 0.0212.

(b) The amount of tip the waiter earns on the best 1​% of such​ weekends is $610.67.

Explanation:

According to the Central Limit Theorem if we have a population with mean μ and standard deviation σ and we take appropriately huge random samples (n ≥ 30) from the population with replacement, then the distribution of the sum of values of X, i.e ∑X, will be approximately normally distributed.

Then, the mean of the distribution of the sum of values of X is given by,

`\mu_(X)=n\mu`

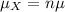
And the standard deviation of the distribution of the sum of values of X is given by,

`\sigma_(X)=√(n)\sigma`

The random variable X can be defined as the tips he receiver per order.

The average tip received by the waiter is, μ = $10.50.

The standard deviation of the tip received by the waiter is, σ = $5.20.

The waiter usually waits on about n = 50 parties over a weekend of work.

So, the distribution of the total tip earned by the waiter is:

`\sum X\sim N(\$525,\ \$36.77)`.

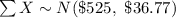(a)

Compute the probability that he will earn at least ​$600 as follows:

`P(\sum X\geq 600)=P(\sum X>600-0.5)`

`=P(\sum X>599.5)\\`

`=P((\sum X-\mu_(X))/(\sigma_(X))>(599.5-525)/(36.77))`

`=P(Z>2.03)\\=1-(Z<2.03)\\=1-0.97882\\=0.02118\\\approx0.0212`

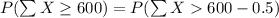
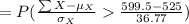
*Use a z-table for the probability.

Thus, the probability that he will earn at least ​$600 is 0.0212.

(b)

Let be a be the amount of tip the waiter earns on the best 1​% of such​ weekends.

That is,

P (∑X > a) = 0.01

⇒ P (∑X < a) = 0.99

⇒ P (Z < z) = 0.99

The value of z for the above probability is:

z = 2.33.

Compute the value of a as follows:

`z=(a-\mu_(X))/(\sigma_(X))`

`2.33=(a-525)/(36.77)`

`a=525+(2.33* 36.77}\\a=610.6741\\a\approx610.67`

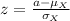
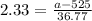
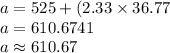
Thus, the amount of tip the waiter earns on the best 1​% of such​ weekends is $610.67.